Find out how to calculate and accurately position increases and decreases
When to use this method
This method of calculating where to position increases and decreases can be used with:
- underarm seams
- V-necks
- raglans
- darts
- button hole placement
- skirt flares
- bias knitting
- gathered sleeves
- circular yokes
If you need to increase (or decrease) a specific number of stitches within a specific number of rows there is a reliable way to calculate this.
The spacing of the increases (ie the number of rows between the increases) is just as important as the number of increases. The last increase should not be right at the end of the required rows as you need to allow for any further shaping. For example your next step could be to cast off for the underarm of a sleeve. You need to consider both the bands of rows between the increases as well as the row which has the increases.
Try this example
| Number of rows available to make all increases | 90 |
| Number of stitches to begin | 70 |
| Number of stitches required at end | 100 |
| Total number of extra stitches required | 30 |
| Number of paired increases (1 at each end of the row) | 15 |
| But to allow for straight rows after the final increase is made + 1 to the total number of pairs | 16 |
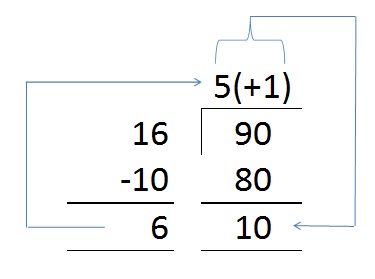
Work through the calculation
Use the figures and formula above and work through the calculation, looking carefully at the arrows to see how the parts of the formula are related.
- You take the number of rows available (90) and divide them by the total of paired increases, 15 plus 1 (16 )
- 16 goes into 90, 5 times plus a decimal point but round this up and show it as (+1) – you can’t have parts of rows! (So 16 times 5 equals 80)
- 90 – 80 leaves 10; now subtract 10 from the numbers of paired increases plus 1 which gives 6
These results are read as follows:-
- Increase every 5 rows 6 times (which gives you 12 stitches and 30 rows)
- Increase every 6 rows 9 times for a total of 15 paired increases plus 1 more band of rows (which gives you 18 stitches and 54 rows plus extra 6 rows)
The method described above was included in Japanese diagrammatic patterns for machine knitting and gained prominence in the 1970s but the equation itself was derived by the ancient Greek mathematician, Diophantos. This method of calculation was called the Magic Formula by an American machine knitting authority because it proved so useful to British and American machine knitters at the time. It is also known as the line sum formula.

